
this graphic shows the value for all trig functions at given angle. I found an amazing applet call the Unit Circle. One other thing a I might want to bring their attention to would be the fact that only two parameters are necessary for specifying a polygon the first line segment (a length) and a number of vertices (considering that the orientation is arbitrary). One might also take a minute to point out that no matter how large or in what orientation the shape finds itself the angles will remain fixed.
when i made my regular polygon i noticed that one thing i might point out about it while students use it would be the linear relationship between side length and parameter and the quadratic relationship it has with area. I am completely new to stuff like this and i have to say playing around with this app is fun. I am actually starting a lesson on slope-intercept form with my class tomorrow and I am excited to share this applet with my students. The applet I made shows a slope-intercept form of an equation and how it changes when the slope and the y-intercept changes. It's more interesting since it uses a picture to show a guy shooting a basketball into the hoop and the user has to figure out the equation so that the basketball will make it into the hoop. The applet helps you to visualize a graph of a quadratic equation by changing the values of a, h, and k. It uses Dan Meyer's Basketball Three-Act and used it in GeoGebra. One of the applets I came across was basketball take 1,2,3,4. I ended up spending a lot of time looking through the applets made by others and just played around with each one. Also, when creating a regular shape on the coordinate plane, students can see the change in values on slope and the y-intercept. Students can also make the connection of making regular polygons using circles and this can lead into a topic of tangent lines and it's angles. Students can also compare a regular polygon with a non-regular polygon and write down similarities and differences between the two (including sum of the interior/exterior angles). Students will be able to find a formula that shows the sum of all interior angles in a regular polygon and also measure of one angle in a regular polygon. They can explore the sides and angles (interior and exterior). Regular shapes - Students will understand the true meaning of a 'regular' shape. GeoGebraTube could have been very helpful if I knew about it. My students and I ended up having a day of exploring instead. I did try using GeoGebra one time couple years ago in my class but had a tough time trying to do some of things I wanted it to show.
#Create regular hexagon gsp5 software#
It's nice that it is a free software for anyone to download. I am also familiar with Geometer's Sketchpad from a course I took in college and GeoGebra seems very similar to it. Make and upload an app to GeoGebra Tube and share the link to it. What does the applet model? What would students learn about the properties of what is modeled if they built such an applet themselves? At the community site GeoGebraTube, find a GeoGebra applet that you think would motivate your students.
It can be something students will have to notice themselves, or something you will be able to point out for them once they had that experience. Find three deep ideas that students can start exploring if they make a regular shape in GeoGebra, like you did. In your reply to this task, answer the following: If you are new to dynamic geometry software, there are good step-by-step instructions online, for example: If you are an advanced GeoGebra user or want to play harder, feel free to expand on this task by constructing other interesting objects. Does the shape stay regular? If not, make a new construction that stays regular when you drag its parts around or resize it. Now drag lines and points that form your shape. Make a regular shape using GeoGebra, maybe a square, a hexagon, or an equilateral triangle. For example, if you add a new point exactly at the intersection of two lines, however you move the lines, the point stays attached to that intersection. You can also put constraints on movement.
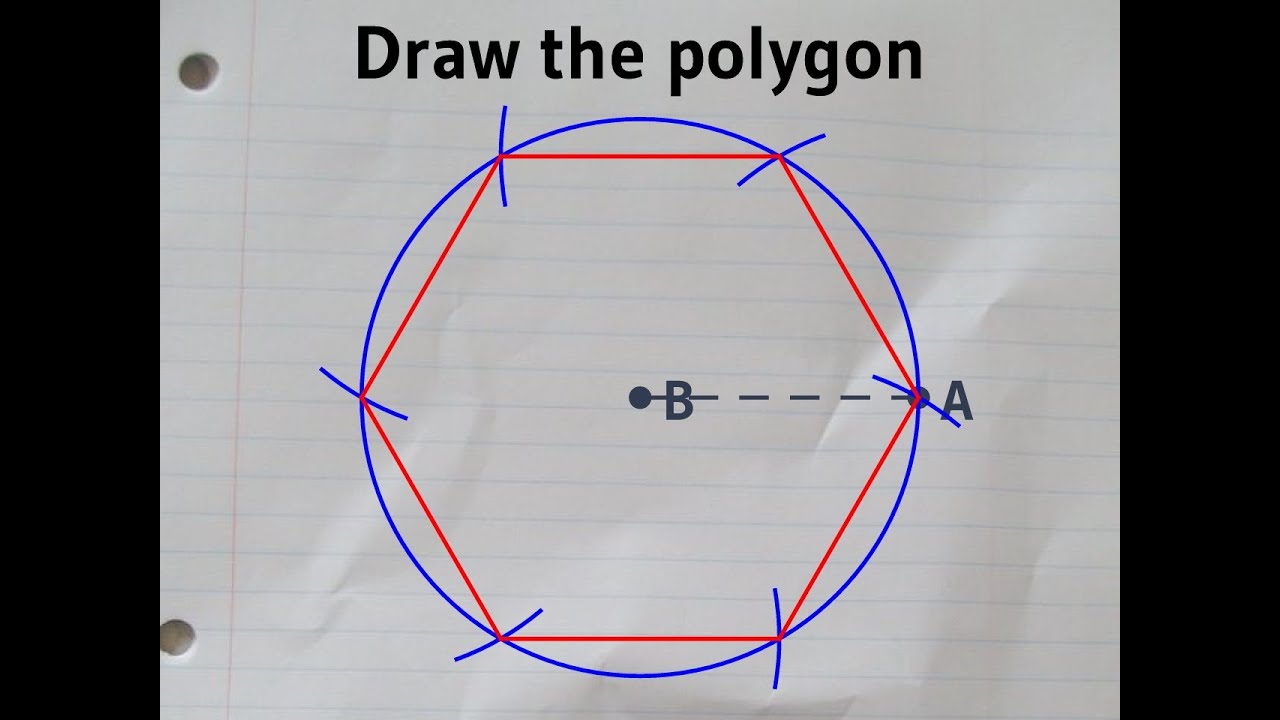
You can move your constructions around and change their shape. Try to draw objects, like points and lines, and make designs out of them. Video: Michael Borcherds, the creator of GeoGebra, talks about computer-based mathematics.ĭownload GeoGebra and play with it for a while. This task introduces GeoGebra - a dynamic geometry + algebra software.


 0 kommentar(er)
0 kommentar(er)
